NÚMEROS TRANSCENDENTES
DOI:
10.47976/RBHM2003v3n575-106Palavras-chave:
SÉTIMO PROBLEMA DE HILBERT, OU PROBLEMA DE HILBERT – EULER, NÚMEROS TRANSCENDENTESResumo
Seja Z o conjunto dos números racionais inteiros e Q o conjunto dos racionais. Seja R o conjunto dos reais e C o dos complexos. Todo número que não é racional chama-se irracional. Seja Qalg o conjunto de todos os números algébricos, i.e., números que são raízes de polinômios p(x) a coeficientes inteiros. A será o conjunto de todos os números algébricos que não são racionais. Um número real ou complexo que não é algébrico chama-se transcendente.
Downloads
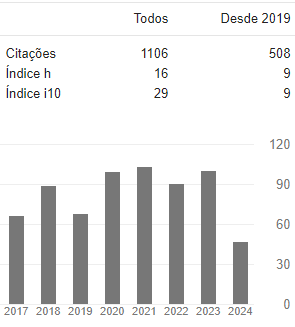
Não há dados estatísticos.
Métricas
Carregando Métricas ...
Referências
[Ad] Adams- Transcendental Number in P-adic Domain, Amer. J. Of Math. 88,279/308.
[Ax] J. Ax, - On Schanuel conjectures, Ann. Of Math.,93 (1971),pp252/268.
[A] N. Allan, -Aproximações por Racionais, Atas do IV Encontro de Brasileiro de Historia da Matemática, Natal, 2001..
[B] A. Baker, -The Theory of Linear Forms and Logarithms, Transcendence Theory: Advances and Applications, Academic Press, NY,1977. P1/28
[Bz] C. Brezinski, -History of Continued Fractions and Padé Approximations, Springer-Verlag, SCM séries, vol 12. NY,1980.
[C] P.L. Cijsous e R. Tijdeman, -On the Transcendence of Certain Power Séries, Acta Arithmetica, 23,1973.
[DF] D. Figueredo, -Números Transcendentes, IMPA, 1964.
[FS] N. I. F el’dman, e A.B. Shidlowskij, -The Development and Present State of the Theory of Transcendental Numbers, Russian Mathematical Notes, 22-3,p1/79.
[G] A. Gelfond, - Transcendental and Algebraic Numbers, Dover, NY, 1960.
[HW] G.H. Hardy e E.M. Wright, -An Introduction to the Theory of Numbers, Oxford, Science Publications,5th edition, Oxford, 1996.
[K] J. F. Koksma,- Diophantische Aproximationen, Chelsea, NY, 1953.
[Lq] Y. Lequain, -Aproximação de um Numero Real por Números Racionais, 19 Colóquio Brasileiro de Matemática, IMPA, 1994.
[L1] S. Lang,-Diophantine Approximations, 5 Colóquio Brasileiro de Matemática, IMPA, 1965.
[L2] S. Lang,- Algebra, Addison Wesley, NY, 1965.
[Ax] J. Ax, - On Schanuel conjectures, Ann. Of Math.,93 (1971),pp252/268.
[A] N. Allan, -Aproximações por Racionais, Atas do IV Encontro de Brasileiro de Historia da Matemática, Natal, 2001..
[B] A. Baker, -The Theory of Linear Forms and Logarithms, Transcendence Theory: Advances and Applications, Academic Press, NY,1977. P1/28
[Bz] C. Brezinski, -History of Continued Fractions and Padé Approximations, Springer-Verlag, SCM séries, vol 12. NY,1980.
[C] P.L. Cijsous e R. Tijdeman, -On the Transcendence of Certain Power Séries, Acta Arithmetica, 23,1973.
[DF] D. Figueredo, -Números Transcendentes, IMPA, 1964.
[FS] N. I. F el’dman, e A.B. Shidlowskij, -The Development and Present State of the Theory of Transcendental Numbers, Russian Mathematical Notes, 22-3,p1/79.
[G] A. Gelfond, - Transcendental and Algebraic Numbers, Dover, NY, 1960.
[HW] G.H. Hardy e E.M. Wright, -An Introduction to the Theory of Numbers, Oxford, Science Publications,5th edition, Oxford, 1996.
[K] J. F. Koksma,- Diophantische Aproximationen, Chelsea, NY, 1953.
[Lq] Y. Lequain, -Aproximação de um Numero Real por Números Racionais, 19 Colóquio Brasileiro de Matemática, IMPA, 1994.
[L1] S. Lang,-Diophantine Approximations, 5 Colóquio Brasileiro de Matemática, IMPA, 1965.
[L2] S. Lang,- Algebra, Addison Wesley, NY, 1965.
Downloads
Publicado
05-11-2020
Métricas
Visualizações do artigo: 579 PDF downloads: 466
Como Citar
SILVA ALLAN, Nelo da. NÚMEROS TRANSCENDENTES. Revista Brasileira de História da Matemática, São Paulo, v. 3, n. 5, p. 75–106, 2020. DOI: 10.47976/RBHM2003v3n575-106. Disponível em: https://mail.rbhm.org.br/index.php/RBHM/article/view/272. Acesso em: 26 abr. 2025.
Edição
Seção
Artigos