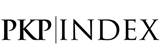Estudo Epistemológico das Desigualdades Matemáticas
DOI:
10.47976/RBHM2022v22n4368-101Palavras-chave:
Desigualdades, Epistemologia, Dialética.Resumo
Las desigualdades han desmostrado ser unas de las herramientas básicas para enfrentar múltiples problemas teórico-prácticos de la ciencia y la tecnología. En este artículo, guiados por la Teoría Dialéctica del Conocimiento, se realiza un estudio epistemológico de las condiciones de evolución y desarrollo de las desigualdades matemáticas, teniendo en cuenta su origen, sistematización y formalización.
Downloads
Métricas
Referências
ABDULLATIF, M. (2011). Al-Battani contributions in Astronomy and Mathematics. En A. O. Shuriye & W. F. Faris (Eds.), Contributions of Early Muslim Scientist to Engineering Studies and Related Sciences (pp. 45-48). Malaysia: IIUM PRESS.
ARGUEDAS, V. (2010). La influencia de la matemática persa en el medioevo. Revista digital Matemática, Educación e Internet, 11(1).
ASHBAUGH, M., BENGURIA, F. (2010). From Carthage to the World: The Isoperimetric Problem of Queen Dido and its Mathematical Ramifications. Queen Dido Conference. Conferencia realizada en Cártago, Tunez, 24-29 de mayo 2010.
BAGNI, G. T. (2005a). Inequalities and Equations: History and Didactics. En M. Bosch, (Ed.). Proceedings of CERME-4 (652-662). Sant Feliu de Guíxols, España: Fundemi IQS-Universitat Ramon Llull.
BAGNI, G. T. (2005b). Equazioni e disequazioni. Riferimenti storici e proprietàinternazionali. La matematica e la sua didattica, 3, 285-296.
BAZZINI, L., TSAMIR, P. (2004). Algebraic equations and inequalities: Issues for research and teaching. Proceedings of the 28th Conference of the International Group of Psychology of Mathematics Education, Bergen, Norway, 1, 137-139.
BLUM-SMIT, B., COSKEY, S. (2017). The Fundamental Theorem on Symmetric Polynomials: History’s First Whiff of Galois Theory. The College Mathematics Journal, 48(1), 18-29.
BOLTIANSKI, V. G. (1981). Lecciones Populares de Matemáticas: Figuras equivalentes y equicompuestas. Moscú, Rusia: Editorial Mir.
BOYER, C. (1968). A history of Mathematics. Nueva York: John Wiley & Sons.
BROUSSEAU, G. (1998). Théorie des situations didactiques. Grenoble: La Pensée Sauvage.
CAJORI, F. (1928). A history of Mathematical Notations, Vol. 1. London. The Open Court Company Publishers.
COLLETTE, J. P. (1986). Historia de las Matemáticas Tomo I. Madrid, España: Siglo XXI España Editores.
CORNU, B. (1991). Limits. In Tall, D. (Ed.). Advanced Mathematical Thinking, 65-81. Dordrecht, the Nederlands: Kluwer Academic Publishers.
COTTLE, R. (2012). William Karush and the KKT Theorem. Documenta Mathematica, Volumen Extra (20012), 255-269.
DEBNATH, LOKENATH (2010), The Legacy of Leonhard Euler: A Tricentennial Tribute, World Scientific,
DIEUDONNÉ, J. (1996). L’Analyse mathématique aux dix-huitième siècle. En Autor (Ed.), Abregé d’Histoire des Mathématiques (pp. 19-53). París, Francia: Hermann Editeurs des Sciences et des Arts.
DREYFUS, T., HOCH, M. (2004) Equations – a structural approach. Proceedings of the 28th Conference of the International Group of Psychology of Mathematics Education, Bergen, Norway, 1, 152-155.
EDWARDS, C. (1979). The historical development of the calculus. New York: Springer-Verlag.
EULER, L. (1767). Solutio facilis problematum quorumdam geometricorum difficillimorum. Novi Commentarii academiae scientiarum Petropolitanae 11, 103-123.
EVES, H. (1997).: Introduçao à História da Matemática. UNICAMP.
FILLOY, E. (1999). Aspectos teóricos del álgebra educativa. México: Grupo Editorial Iberoamericana
FINK, A. M. (2000). An Essay on the History of Inequalities. Journal of Mathematical Analysis and Applications, 249, 118–134.
FOWLER, D., ROBSON, E. (1998). Square Root Approximations in Old Babylonian Mathematics: YBC 7289 in context. Historia Mathematica, 25, 366–378.
GHEVERGHESE, G. (2008). A Brief History of Zero. Iranian Journal for the History of Science, 37-48
GIOVANNINI, E. (2015). David Hilbert y los Fundamentos de la Geometría (1891-1905). En S. Rahman & J. Redmond (Eds.). Cuadernos de lógica, epistemología y lenguaje, (Vol. 8). Berkeley, California: Individual Author and College Publications.
GIOVANNINI, E. (2017). El reto de Hilbert en la teoría de las magnitudes poligonales: Un capítulo en la axiomatización sintética de la geometría euclidiana. Revista latinoamericana de filosofía, 43(2), 207-235.
GONZÁLEZ, F. A., MARTÍN-LOECHES, M., SILVÁN, E. (2010). Prehistoria de la matemática y mente moderna: pensamiento matemático y recursividad en el paleolítico franco-cantábrico. Dynamis, 30, 167-195.
GONZÁLEZ, J., MORALES, A., SIGARRETA, J. M. (2013). Concepciones sobre el infinito: Un estudio a nivel universitario. Revista Digital Matemática, Educación e Internet, 3(2), 1-12.
GONZÁLEZ, J. (2016). Geometría, una reflexión infinita (Tomo I). Ed. Universidad Autónoma de Guerrero. Gro. Méx.
HALMAGHI, E., LILJEDAHL, P. (2015). Inequalities in the History of Mathematics: From Peculiarities to a Hard Discipline. GeoGebra International Journal of Romania, 4(2), 43–56.
HANS, J., MUÑOZ, J., FERNÁNDEZ, A. (2011). Puzles de cuadraturas. Revista Suma+, 66, 43-46.
HARDY, G. (1915). Notes on some points in the integral calculus, XLI. On the convergence of certain integrals and series. Messenger of Math, 45, 163–166.
HARDY, G. (1919). Notes on some points in the integral calculus, LI. On Hilbert’s double-series theorem, and some connected theorems concerning the convergence of infinite series and integrals. Messenger of Math, 48, 107–112.
HARDY, G. (1920). Notes on a theorem of Hilbert. Mathematische Zeitschrift, 6, 314–317.
HARDY, G. (1925). Note on a theorem of Hilbert concerning series of positive terms. Proceedings of the London Mathematical Society, 23(2), 45–46.
HARDY, G. H., LITTLEWOOD J. E., POLYA, G. (1934). Inequalities. Cambridge, United Kingdom: Cambridge University Press.
ILLANA, J. (2012). Matemáticas en el antiguo Egipto. Revista Suma+, 71, 47-61.
JORDAN, C. (1893) Cours d’Analysede l’École Polytechnique 2. Ed. Paris. Gauthier-Villars
JORDAN, K. E., Brannon, E. M., Logothetis, N. K., & Ghazanfar, A. A. (2005). Monkeys match the number of voices they hear to the number of faces they see. Journal of Vision, 5(8),887, 887a. doi:10.1167/5.8.887.
JUNGK, W. (1979). Conferencias sobre metodología de la enseñanza de la matemática. Tomo II, 1ª. Parte. La Habana: Editorial Pueblo y Educación
KATZ, V. ( 2009). History of Mathematics. 3ª.ed. Ed. Pearson. New York
KASIR, D. S. (1931). The Algebra of Omar Khayyam. The Mathematics Teacher, 25(4), 238-241.
KAZARINOFF, N. (1961). Geometric inequalities. New Haven, Connecticut: Yale University.
KLINE, M. (1972). Mathematical Thought from Ancient to Modern Times. New York: Oxford University Press.
KOUKI, R. (2008) Enseignement et apprentissage des équations, inéquations et fonctions au secondaire : entre syntaxe et sémantique Université. Tesis Doctoral (no publicada). Universidad Claude Bernard Lyon 1
KUFNER, A., MALIGRANDA, L., PERSSON, L. E. (2006). The prehistory of the Hardy inequality. American Mathematical Monthly, 113(8),715–732.
LAGRANGE, J. L. (1769). Sur la résolution des equations algebriques. Memoires de l’Académie des Sciences et Belles-Lettres de Berlin. En Oeuvres Completes de Lagrange Tomo II (540-578). Paris: Gauthier-Villars.
LANDAU, E. (1921). Letter to G. H. Hardy, June 21, 1921.
LANDAU, E. (1924). Letter to G. H. Hardy, December 13, 1924.
LANDAU, E., Shur, I., & Hardy, G. H. (1926). A note on a theorem concerning series of positive terms: Extract from a letter. Journal of the London Mathematical Society, 1, 38–39. doi:10.1112/jlms/s1-1.1.38
LENIN, V. I. (1979). Cuadernos Filosóficos (p. 165). La Habana: Editora Política.
LINCHEVSKI, L., & Sfard, A. (1991). Rules without reasons as processes without objects – The case of equations and inequalities. Proceedings of PME15, Assisi, Italy, 2, 317-324.
MAKAROV, B. M., GOLUZINA, M. G., LODKIN, A. A., PODKORYTOV, A. N. (1992). Selected Problems in Real Analysis. Translations of Mathematical Monographs. EEUU: American Mathematical Society.
PARRA, E. (2009). Arquímedes: su vida, obras y aportes a la matemática moderna. Revista digital Matemática, Educación e Internet, 9(1), 1-40.
PEANO, G. (1887) Applicazioni Geometriche. Torino. Fratelli Bocca Editori
PÉREZ, M. A. (2009). Una Historia de las Matemáticas: Retos y conquistas a través de sus personajes (p. 33). Madrid, España: Editorial Visión Libros.
PÉREZ R., BERENGUER I., COBO, B., DAZA, D., FERNÁNDEZ, F., POSADAS, M., PAYÁ, A. (2000). Isoperímetros en la Grecia Antigua. Revista Suma, 34, 95-98.
RIESZ, M. (1923). Letter to G. H. Hardy, 1919 or 1920.
RUIZ, F. (2015). Algunos problemas de optimización geométrica. Pensamiento Matemático, 5(2), 027-054.
SACKUR, K. (2004). Problems related to the use of graphs in solving inequalities. Proceedings of the 28th Conference of the International Group of Psychology of Mathematics Education, Bergen, Norway, 1, 148-152.
SÁNCHEZ, A., SIGARRETA, J. (2011). Estudio epistemológico de las geometrías no-euclidianas. Matemáticas: Enseñanza Universitaria, 9(2), 117-132.
SCHUR, I. (1911). Bemerkungen zur Theorie der beschränkten Bilinearformen mit unendlich vielen Veränderlichen. Journal für die reine und angewandte Mathematik, 1911(140), 1–28.
SCHUR, I. (1919). Letter to G. H. Hardy, 1918 or 1919.
SOLAECHE, M. C. (2002). Omar Khayyam: las Matemáticas, la Nada, el Vino, el Torno y la Amada. Divulgaciones Matemáticas, 10(1), 79–83.
STEEL, J. M. (2004). The Cauchy-Schwartz master class. An introduction to the art of Mathematical Inequalities. Inglaterra: Cambridge University Press.
STEWART, I. (2008). Historia de las matemáticas en los últimos 10000 años. Barcelona, España: Editorial Crítica.
TALL, D. (2004). Reflections on research and teaching of equations and inequalities. Proceedings of the 28th Conference of the International Group of Psychology of Mathematics Education, Bergen, Norway, 1, 158-161.
TALL, D., VINNER, S.: 1981, Concept image and concept definition in Mathematicswith particular reference to limits and continuity, Educational Studies in Mathematics, 12, 151-69.
TSAMIR, P., TIROSH, D., TIANO, S. (2004). New errors and old errors: The case of quadratic inequalities. Proceedings of the 28th Conference of the International Group of Psychology of Mathematics Education, Bergen, Norway, 1, 155-158.
VERA, F. (1970). Científicos Griegos Tomo I. Madrid, España: Aguilar S. A. de Ediciones.
VER EECKE, P. (1959) Diophante d’Alexandrie. Les Six Livres Arithmétiques et le Livre des Nombres Polygones. París : Albert Blanchard
VIRGILIO, P. (1890). La Eneida. Madrid, España: Editorial Librería Viuda de Hernando y Compañía.
Downloads
Publicado
Métricas
Visualizações do artigo: 751 PDF downloads: 539